Andrew Ranicki recently asked me to compute the Hirzebruch L-polynomial L₈ expressing the signature of a 32-dimensional manifold in terms of its Pontrjagin numbers. (Hirzebruch’s book Topological Methods in Algebraic Geometry only lists L₁ through L₅, in §1.5.)

Here is Mathematica code to compute it, or any other member of a multiplicative sequence of polynomials:

 K[Q_,n_Integer] := Module[{z,x},
   SymmetricReduction[
      SeriesCoefficient[
       Product[ComposeSeries[Series[Q[z],{z,0,n}], 
         Series[x[i]z,{z,0,n}]],{i,1,n}],n], 
      Table[x[i],{i,1,n}], 
      Table[Subscript[c,i],{i,1,n}]][[1]] // FactorTerms]

The generating series for the L-sequence is $\frac{\sqrt{z}}{\tanh\sqrt{z}}$ so L₈ can then be computed by running:

  K[ Sqrt[#]/Tanh[Sqrt[#]]&, 8] /. c->p

which produces:

Some other L-polynomials (now published as OEIS sequence A237111):

The last question of my differential equations exam yesterday was:

My favorite answer to it—one which followed a rigorous & correct mathematical analysis—was:

[The question, incidentally, made the most oblique of references to Buñuel’s Obscure Object of Desire (1977).]

I recently gave a short talk about Dürer’s engraving Melencolia I (1514) in Rochelle Tobias’s seminar in the German dept at Hopkins.

To begin I talked a little about the magic square of numbers. First of all it’s “normal”: each number 1,…,16 appears once. And not only do the rows, columns and two diagonals sum to 34, so do the 4 corners, the 4 numbers in the center, and the remaining parallel segments. The bottom row serves as Dürer’s second signature: the middle two numbers form the year the engraving was made and the outer numbers correspond to Dürer’s initials A=1, D=4. It’s believed that the sum 34 refers to Dürer’s age when he engraved it, 43, reinforced by the square’s placement beside the hourglass. There was a popular magical/astrological association at the time between magic squares and planets. Saturn corresponded to 3×3 magic squares and Jupiter to 4×4. Rochelle pointed out that it makes sense for Dürer to have used a 4×4 rather than a 3×3 (Saturn is closely associated with melancholy) since things associated with Jupiter were supposed to help fend off melancholy.

The Solid

The solid in the engraving is rather mysterious and was the main subject of my talk. People have been debating it for at least the last 100 years with a possible breakthrough in 2004. The most influential work on the engraving—Raymond Klibansky’s magnum opus Saturn and Melancholy (1964)—has the least to say about the solid of all the objects in the engraving.

The solid is completely unique to the engraving and doesn’t appear anywhere else in art or mathematics. At first it was thought to simply be a cube with two edges “truncated”, that is chopped off, leaving behind triangular faces. It doesn’t quite look like a cube but it was thought that Dürer simply made a mistake with perspective. Eventually it was agreed that Dürer wasn’t the sort to make mistakes with perspective and hence that the underlying shape must not be a cube. There have been quite a few papers over the years correcting for perspective in various ways to compute what the angles of the solid are, reaching different conclusions.

The papers which most interested me were recent ones inspired by a paper of JV Field at Birkbeck:

Rediscovering the Archimedean Polyhedra: Piero della Francesca, Luca Pacioli, Leonardo da Vinci, Albrecht Dürer, Daniele Barbaro, and Johannes Kepler, Archive for History of Exact Sciences, Vol. 50, No. 3/4 (September 1997), pp. 241-289.

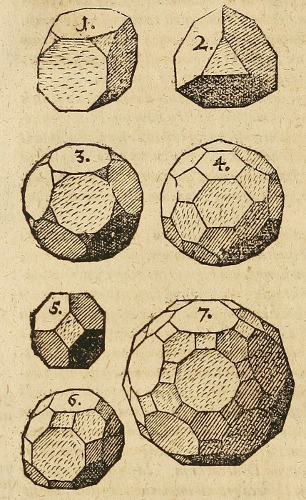
I probably found that paper the most interesting of the lot. It’s on the history of Archimedean solids. The simplest solids of all are the Platonic solids, solids like the cube whose faces are all the same shape and size. 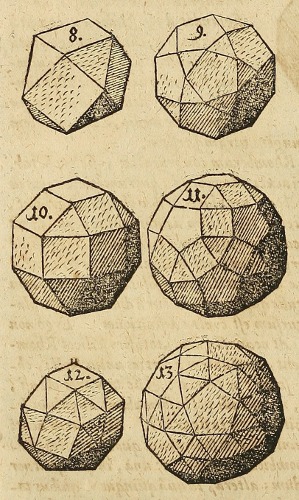The ancient Greeks showed that there are just 5 of them—the tetrahedron, the hexahedron (=cube), the octahedron, the dodecahedron, and the icosahedron—and they used them as the basis for theories about the physical universe. The next simplest solids are the Archimedean solids, solids whose vertices are all “the same” but whose faces are not. The ancient Greeks determined that there are just 13 of them (or 15 depending on how you count—2 of the 13 differ from their mirror images). One of the last great ancient Greek mathematicians Pappus listed all 13 of them, credited their discovery to Archimedes, but instead of drawing them cryptically wrote the total number of triangles, squares, pentagons,… each had. His work was correct but apparently forgotten—for more than a thousand years—until in 1619 Johannes Kepler published a complete list including drawings of each (reproduced above).

In the meantime though quite a few Renaissance artists unaware of Pappus’s work had had a go at constructing them, among them Dürer. There’s a great table in Field’s paper listing which of the solids each of four artists is known to have constructed:

The table suggests that Dürer discovered two Archimedean solids previously unknown to Renaissance artists, the truncated cuboctahedron (6) and the snub cube (12). Dürer worked in two different ways: By drawing projections onto the three coordinate planes and by making what he called a Netz—lines on paper you can cut and fold along to make a model of a solid. Dürer appears to have invented this method actually, and he used it to discover new solids. 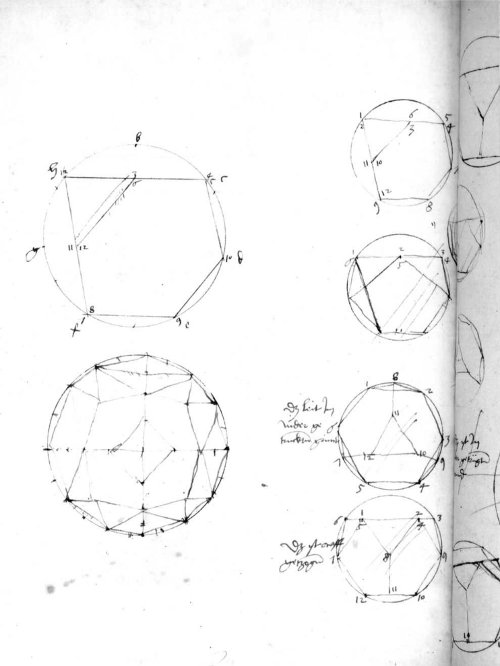Field thinks Dürer may have been aware of all 13 solids since one can construct a Netz for each of the missing solids by small modifications to the new Netze Dürer discovered, but that Dürer didn’t write them all down because Dürer didn’t realize there are only 13 of them. Field speculates that Kepler may have learned all this from Dürer and synthesized it with the work of Pappus.

To cut to the chase, this chap Hans Weitzel found a sketch by Dürer in a library in Nürnberg in 2003 where Dürer had constructed two known Archimedean solids but on the same page had made an enigmatic drawing of a pentagon inscribed in a circle:

A further hypothesis on the polyhedron of A. Dürer’s engraving Melencolia I, Historia Mathematica, Vol. 31, Issue 1, Feb 2004, pp.  11–14.

Weitzel argues that Dürer was on that page trying to construct a new Archimedean solid which doesn’t exist (one where each vertex touches one triangle and two pentagons) and that the pentagon Dürer in the process drew exactly matches the face of the solid in the engraving. In fact Weitzel finds that the angles of the sketched pentagon agree with the corrected angles computed by others for the mystery solid. So Weitzel argues that the solid in the engraving is the result of a failed attempt by Dürer to discover a new Archimedean solid.

This seems consistent with Erwin Panofsky’s interpretation of the engraving as a spiritual self-portrait of Dürer, exploring the relationship between creative genius and melancholy. That’s the thrust of it as I understand it anyway. It’s kind of intense. That’s not even getting into black bile, the four temperaments, and the rest of it.

Saturn and Krypton—Superman as Winged Melancholia?

A week or so after my talk it suddenly struck me that Dürer’s pentagon is quite similar to the outline of Superman’s “S” shield.

This points to remarkable parallels between Melencolia I and the Superman universe.

Compare, for example, the effect of Dürer’s solid on Winged Melancholia to the effect of the Kryptonite solid on the flying figure in the panel at the far right.

Janine’s Paper Universe

One of my favorite passages from WG Sebald’s Rings of Saturn (1995) alludes to Dürer’s engraving:

“Many a time, at the end of a working day, Janine would talk to me about Flaubert’s view of the world, in her office where there were such quantities of lecture notes, letters and other documents lying around that it was like standing amidst a flood of paper. On the desk, which was both the origin and the focal point of this amazing profusion of paper, a virtual paper landscape had come into being in the course of time, with mountains and valleys. Like a glacier when it reaches the sea, it had broken off at the edges and established new deposits all around on the floor, which in turn were advancing imperceptibly towards the centre of the room. Years ago, Janine had been obliged by the ever-increasing masses of paper on her desk to bring further tables into use, and these tables, where similar processes of accretion had subsequently taken place, represented later epochs, so to speak, in the evolution of Janine’s paper universe. The carpet, too, had long since vanished beneath several inches of paper; indeed, the paper had begun climbing from the floor, on which, year after year, it had settled, and was now up the walls as high as the top of the door frame, page upon page of memoranda and notes pinned up in multiple layers, all of them by just one corner. Wherever it was possible there were piles of papers on the books on her shelves as well. It once occurred to me that at dusk, when all of this paper seemed to gather into itself the pallor of the fading light, it was like the snow in the fields, long ago, beneath the ink-black sky. In the end Janine was reduced to working from an easychair drawn more or less into the middle of her room where, if one passed her door, which was always ajar, she could be seen bent almost double scribbling on a pad on her knees or sometimes just lost in thought. Once when I remarked that sitting there amidst her papers she resembled the angel in Dürer’s Melancholia, steadfast among the instruments of destruction, her response was that the apparent chaos surrounding her represented in reality a perfect kind of order, or an order which at least tended towards perfection. And the fact was that whatever she might be looking for amongst her papers or her books, or in her head, she was generally able to find right away.”

«Oft, zu Ende des Tages, habe ich mich mit Janine über die Weltauffassung Flauberts unterhalten in ihrem Büro, in dem solche Mengen von Vorlesungsnotizen, Briefen und Schriftsstücken jeder Art herumlagen, daß man meinte, mitten in einer Papierflut zu stehen. Auf dem Schreibtisch, dem ursprünglichen Ausgangs- beziehungsweise dem Sammelpunkt der wundersamen Papiervermehrung, war im Verlaufe der Zeit eine richtige Papierlandschaft mit Bergen und Tälern entstanden, die inzwischen an den Rändern, so wie ein Gletscher, wenn er das Meer erreicht, abbrach und auf dem Fußboden ringsum neue, ihrerseits unmerklich gegen die Mitte des Raumes sich bewegende Ablagerungen bildete. Vor Jahren bereits war Janine von den immerzu weiterwachsenden Papiermassen auf ihrem Schreibtisch gezwungen gewesen, an andere Tische auszuweichen. Diese Tische, auf denen sich in der Folge ähnliche Akkumulationsprozesse vollzogen hatten, repräsentierten sozusagen spätere Zeitalter in der Entwicklung des Papieruniversums Janines. Auch der Teppich war seit langem schon unter mehreren Lagen Papier verschwunden, ja das Papier hatte aufgefangen, vom Boden, auf den es fortwährend aus halber Höhe hinabsank, wieder die Wände emporzusteigen, die bis zum oberen Türrand bedeckt waren mit einzelnen, jeweils nur an einer Ecke mit einem Reißnagel befestigten, teilweise dicht übereinandergehefteten Papierbögen und Dokumenten. Auch auf den Büchern in den Regal dieses Papier versammelte auf sich in der Stunde der Dämmerung den Widerschein des vergehenden Lichts, wie vordem, so habe ich mir einmal gedacht, unter dem tintenfarbenen Nachthimmel der Schnee auf den Feldern. Janines letzter Arbeitsplatz ist ein mehr oder weniger in die Mitte ihres Büros gerückter Sessel gewesen, auf dem man sie, wenn man an ihrer stets offenen Tür vorbeikam, sitzen sah, entweder vornübergebeugt kritzelnd auf einer Schreibunterlage, die sie auf den Knien hielt, oder zurückgelehnt und in Gedanken verloren. Als ich gelegentlich zu ihr sagte, sie gleiche, zwischen ihren Papieren, dem bewegungslos unter den Werkzeugen der Zerstörung verharrenden Engel der Dürerschen Melancholie, da antwortete sie mir, daß die scheinbare Unordnung in ihren Dingen in Wahrheit so etwas wie eine vollendete oder doch der Vollendung zustrebende Ordnung darstelle. Und tatsächlich wußste sie, was immer sie in ihren Papieren, in ihren Büchern oder in ihrem Kopf suchte, in der Regel auf Anhieb zu finden.»

References

• Johannes Kepler, Harmonices Mundi libri V, Linz, 1619.
• Erwin Panofsky, The Life and Art of Albrecht Dürer, Princeton, 1955.
• Raymond Klibansky, Erwin Panofsky & Fritz Saxl. Saturn and Melancholy. Studies in the History of Natural Philosophy, Religion, and Art, London, 1964.
• Klaus-Peter Schuster, Melencolia I. Dürers Denkbild. Dissertation. Universität Göttingen 1975. Berlin 1991.
• Terence Lynch, The Geometric Body in Dürer’s Engraving Melencolia I, Journal of the Warburg and Courtauld Institutes, Vol. 45, (1982), pp. 226-232.
• JV Field. Rediscovering the Archimedean Polyhedra: Piero della Francesca, Luca Pacioli, Leonardo da Vinci, Albrecht Dürer, Daniele Barbaro, and Johannes Kepler. Archive for History of Exact Sciences, Vol. 50, No. 3/4 (September 1997), pp. 241-289.
• Peter Schreiber, A New Hypothesis on Dürer’s Enigmatic Polyhedron in His Copper Engraving “Melencolia I”, Historia Mathematica, Vol. 26, Issue 4, Nov 1999, pp. 369–377.
• Hans Weitzel, A further hypothesis on the polyhedron of A. Dürer’s engraving Melencolia I, Historia Mathematica, Vol. 31, Issue 1, Feb 2004, pp.  11–14.
• Hans Weitzel, Zum Polyeder auf A. Dürers Stich Melencolia I — ein Nürnberger Skizzenblatt mit Darstellungen archimedischer Körper, Sudhoffs Archiv, Bd. 91, H. 2 (2007), pp. 129–173.

My toast to my sister at her wedding

I’m not very good at speaking in public. I mean, it’s my job—I’m a university lecturer—but that’s different. There’s a blackboard and if I speak into it then the sound waves reflect to the audience, and if I wait long enough then by the time I turn round everyone’s gone.

When I started thinking about giving a wedding toast, I thought of something a friend of mine, a very grand man, the Professor of Algebraic Surgery at the University of Edinburgh, once told me about his father’s wedding. His father is the German literary critic Marcel Reich-Ranicki. Most of you have probably never heard of him, but in Germany he’s a household name. Think of him as the German Harold Bloom, except that in Germany, as hard as it may be to believe, literary critics are A-list celebrities.

In 1940 Reich-Ranicki and his entire family found themselves in the Warsaw Ghetto. Two years later he learned that his life would be spared but his fiancée’s would not, unless he married her immediately. At the hastily organized wedding, he was suddenly struck by the words:

Ward je in dieser Laun’ ein Weib gefreit?

That’s an archaic German translation of a line from Shakespeare’s Richard III:

Was ever woman in this humour wooed?

A recent BBC radio program began with a speech by Reich-Ranicki about his wedding, and went on to explore Shakespeare’s ability to speak across so many cultures and ages. That made me wonder what Shakespeare might have to say to us on this occasion.

Unfortunately I’m no literary genius so the first words which struck me were:

To be, or not to be

Seeing my little sister get married before me does affect my outlook in various ways. But it doesn’t throw me into existential angst. Even if my parents wish it would.

Actually, the reason I thought of that quotation first was because in thinking about Nazis and Shakespeare, I immediately thought of Ernst Lubitsch’s 1942 film To Be or Not to Be. It’s about a troupe of actors in Nazi-occupied Warsaw who use disguise and acting to fool the occupying troops. Jack Benny plays a Shakespearean actor whose wife instructs her secret lover to wait in the audience until Benny begins Hamlet’s famous soliloquy, at which point he is to walk out and come to her dressing room, as this will assure their privacy. The scene recurs throughout the film with a very funny twist in the final scene, which I won’t spoil for you. The film is hilarious and clearly influenced Quentin Tarantino’s Inglorious Basterds in many ways. But I digress.

The next Shakespeare quotation I thought of was:

See, how she leans her cheek upon her hand!
O, that I were a glove upon that hand,
That I might touch that cheek!

The bride is indeed exceedingly gorgeous today, but what an incestuous thing for a brother to say in a wedding toast!

Finally I thought of Sonnet 116:

Let me not to the marriage of true minds
Admit impediments. Love is not love
Which alters when it alteration finds,
Or bends with the remover to remove:
O no! it is an ever-fixed mark
That looks on tempests and is never shaken;
It is the star to every wandering bark,
Whose worth’s unknown, although his height be taken.
Love’s not Time’s fool, though rosy lips and cheeks
Within his bending sickle’s compass come:
Love alters not with his brief hours and weeks,
But bears it out even to the edge of doom.
If this be error and upon me proved,
I never writ, nor no man ever loved.

Stunning and very appropriate for this couple today. [And isn’t it wonderful that in Shakespeare’s English, “prove” rhymed with “love”?]

But there is a higher authority than Shakespeare, at least for this particular audience, and I want to close with some of his words.

Love:

Humans do claim a great deal for that particular emotion.

The man who said those words, as many of those gathered here today probably already know, was:

Spock

Stardate 5725.6

To the bride and to love!

I’m exceedingly happy to be joining the topology group at Johns Hopkins University. Please note my new address.