
|
Ripples
by Carl
|

|
Ripples
by Carl
|
“If, in some cataclysm, all of scientific knowledge were to be destroyed, and only one sentence passed on to the next generations of creatures, what statement would contain the most information in the fewest words? I believe it is the atomic hypothesis that all things are made of atoms—little particles that move around in perpetual motion, attracting each other when they are a little distance apart, but repelling upon being squeezed into one another.” —Richard P. Feynman, Feynman Lectures on Physics, 1-2.
To compose this experimental piece, I released particles into a one-dimensional potential field. Actually, I simulated this within a computer by means of a discrete numerical integrator. I interpreted the particles’ positions as pitch, and their velocities as rhythm; roughly speaking, the faster a particle moves, the more frequently its pitch is heard. Since the simulated space is continuous, or at least nearly so, many wonderful microtones emerge.
Listen to how the tense, exotic sonorities relax onto more familiar, consonant ones, at least most of the time. For I designed the potential fields so that the particles would be attracted to locally consonant intervals (cf. the discussion and figure below). So listen for the particles to oscillate around these consonant intervals, accelerating, then decelerating as they change direction like pendulums. Due to a friction term, the particles in fact eventually succumb to the consonant attractors like water swirling down into a drain.
I generated the four movements by subtly altering the potential field. In this way, each movement begins with a “big bang” corresponding to the same initial configuration of twenty equally spaced particles, but after five seconds of silence, the differences in potential fields gradually lead to dramatically divergent long-term behavior.
My favorite parts of the work are the initial maelstrom of the first movement and the emergent tonality of the fourth.
| MP3 | OGG | gzip’d CMIX | |
|---|---|---|---|
| First Movement | 3.7MB | 3.5MB | 108K |
| Second Movement | 3.2MB | 3.1MB | 114K |
| Third Movement | 4.2MB | 3.8MB | 110K |
| Fourth Movement | 3.6MB | 3.3MB | 120K |
On a personal note, I composed the first movement during the first days of the second gulf war. Although I didn’t know anyone directly involved in the conflict, it was nevertheless a very tense time for me. I had nightmares in the green luminescence of night-vision goggles, stumbling through sand, gasping for air through a greasy gas mask as anti-aircraft fire streaked overhead. When I hear the first movement, this is still the image I personally see.
I derived the potential fields from the so-called dissonance curves first introduced by Kameoka and Kuriyagawa in 1969. These authors developed hump-shaped curves resembling chi-squared distributions which approximate the perceived “dissonance” between two pure tones (sin waves) as a function of their frequency difference. The dissonance of a complex tone may be approximated by summing the dissonance between each pair of partials within the tone. If we superpose two harmonic tones (a harmonic tone being the superposition of pure tones whose frequencies are integer multiples of a single base frequency) and approximate their combined dissonance as we vary one of the two base frequencies, then we obtain a complex curve with several minima corresponding roughly to the so-called Just musical intervals (ratios of small integers), because at such intervals two or more of the harmonic tones’ partials coincide. In constructing the potential fields I relied on constants which Sethares obtained by nonlinear approximation of empirical psychoacoustic data.
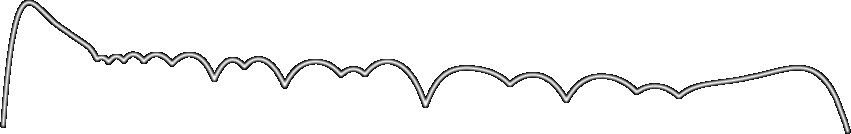 |
|
|
The piece was realized by a custom program written in the purely functional programming language Haskell. This program produced a so-called score file, which was then rendered by the CMIX strum instrument (whose underlying design is due to Kevin Karplus and Alex Strong). The resulting audio file was then compressed with the programs oggenc, lame, and flac. All programs and tools used are free (e.g. gpl’d) software.