A still from Crutchfield’s 1986 film (converted to black and white).
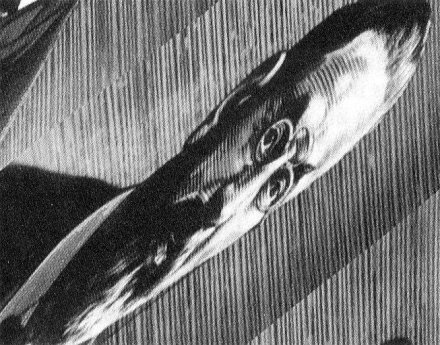
This music was inspired by a short film by physicist Jim Crutchfield which accompanied his 1986 article Chaos in Scientific American. In the film a portrait of French mathematician Henri Poincaré (1854-1912), the father of both algebraic topology and modern dynamical systems theory, is stretched at an irrational angle by Arnold’s cat map (described below). The portrait soon stretches into a noisy bustle of shimmering pixels but at the end of the film Poincaré’s image suddenly reassembles. Crutchfield intended this as a historical joke because Poincaré dispelled a commonly held belief among physicists that ‘noisy’ systems must necessarily destroy information.
The construction of Arnold’s cat map is simple. Because the linear transformation (x,y) → (2x+y, x+y) and its inverse have integer coefficients, they determine an automorphism of the unit torus. (Vladimir Arnold illustrated its effects on a cat's face in his book Problèmes Ergodiques de la Mécanique Classique.) Despite the cat map's apparent simplicity, it exhibits remarkable behavior when iterated. For instance because the linear transformation’s attracting eigenspace has irrational slope (√5-1)/2, the cat map has an attractor wrapping densely through the torus, coming arbitrarily close to every point. Yet at the same time all rational points—also dense in the torus—relax onto (arbitrarily long) periodic orbits.
Like the film that inspired it, Portrait of Poincaré was generated by the cat map. A drone with wonderful, ringing overtones pervades the piece. The piece comes from applying the cat map to this simple drone. How? The notes of the drone are conceived as points of the torus; a point’s x-component determines its position within its rhythmic cell and its y-component its stereo-spread (how far left or right-channel it is). In particular the drone corresponds to the graph of sin(2πt) sampled at 20 evenly spaced points of the unit interval, that is it corresponds to the points:
These points are iterated by the cat map to generate the additional rhythmic cells, each iterate being placed on its own overtone of the drone’s base frequency. This results, quite surprisingly, in almost improvisatory, dance-like rhythms.
This is the first piece to which I have put several new ideas—most notably functions of the interval in conjunction with the Ξ-operator to control dynamics.
| MP3 | OGG | FLAC | gzip’d CMIX |
|---|---|---|---|
| 4.7MB | 4.4MB | 35MB | 108K |
NOTA BENE: This work is licensed under a Creative Commons License, and is Copyright © 2003 by Carl . Please contact me if you would like either to create a derived work, or to use this piece for commercial purposes.
The piece was realized by a custom program written in the purely functional programming language Haskell. This program produced a CMIX score file, which was then rendered by the CMIX strum instrument (whose underlying design is due to Kevin Karplus and Alex Strong). The resulting audio file was then compressed with the programs oggenc, lame, and flac. All programs and tools used are free (e.g. gpl’d) software.